Single Continuous Surface
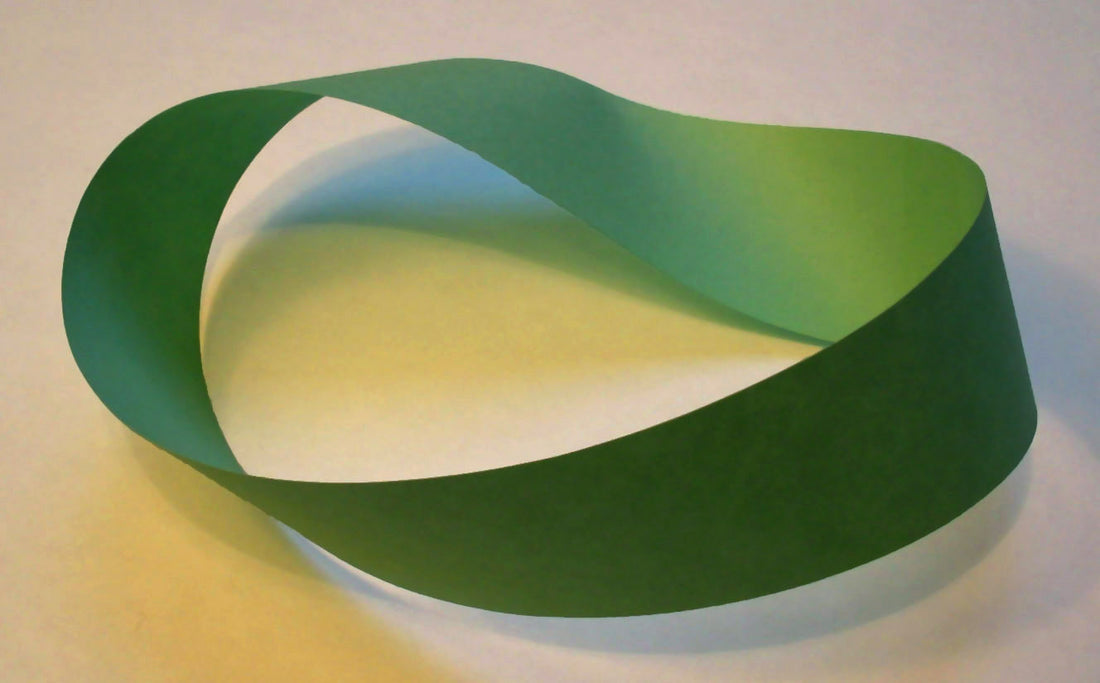
What do the Google Drive logo, old fashioned conveyor belts, and Gabriel Garcìa Màrquez’s novel One Hundred Years of Solitude all have in common? They each pay homage to the Möbius Strip, a single-sided, non-orientable surface.
The Möbius Strip was “discovered” independently and almost simultaneously in 1858 by two German mathematicians: August Ferdinand Möbius and Johann Benedict Listing. It wasn’t the physical object itself that they crafted. Möbius Strips have been spotted in Roman mosaics dating back to 3rd Century CE and have long been used in conveyor belts to even out surface wear and tear. And anyone can make a strip: you simply affix the ends of a rectangular length after giving one end an odd number of twists. Where the two Germans struck mathematical gold was in discovering that the single-surfaced shapes have a set of unique non-orientable properties that would give rise to an entire field of study: topology.
To understand its properties, imagine that you are an ant stuck on a Möbius Strip. If you make one full tour of the closed circuit, you unfortunately won’t make it back to where you started. You’ll be upside down. You’ll need to scuttle around a second time to return, the right way up, to your starting point. Aside from this all making you a very tired ant, you’ll also have lost all sense of orientation.
Topology is the study of properties, like orientability, of geometric objects that are preserved despite continued deformations such as stretching or twisting. This may sound a little bit bland, but topology has very serious real-world applications, like within the world of chemistry. Several chemical compounds have the same structure and can only be distinguished based on their orientability. For example, L-methamphetamine, the ingredient in Vicks Vapor Inhalers, has the same structure as D-methamphetamine, an illegal drug. If it wasn’t for the Möbius Strip and its implications on orientability, who knows what we’d be using the clear our stuffy noses.
Continuous and dynamic, the Möbius Strip is also a never-ending source of inspiration for artists and designers. From the Google Drive logo to the universal symbol for recycling, its loopy-ness suggests a kind of flowing synergy, interminable and reciprocal. It’s not surprising that its mathematical mysteriousness would make the Möbius Strip a favourite of Dutch graphic designer M.C. Escher. His work Mobius Band I (1961) depicts three-folded flatfish biting each other’s tails, whilst Mobius Band II (1963) shows ants crawling around a twisted figure-eight Möbius Strip.
But what if we are like Escher’s ants (or flatfish) and life is just one big Möbius Strip? It’s this non-linear shape of time that Gabriel Garcìa Màrquez toyed with in his masterpiece One Hundred Years of Solitude in which the people of Macondo slide continuously and simultaneously from past, present, and future.
Although the consecutive lockdowns put in place due to the COVID-19 pandemic made life feel like a never-ending loop for many of us, for now the Möbius Strip seems to be purely spatial and (hopefully) not temporal.
_____________________
References
wikipedia.org/wiki/Mobius_strip
smithsonianmag.com/science-nature/mathematical-madness-mobius-strips-and-other-one-sided-objects-180970394/
scientificamerican.com/article/the-timeless-journey-of-the-moebius-strip/
comsol.com/blogs/mobius-strips-where-math-meets-art/
Images
1. Möbius Strip. Image Credit: David Benbennick, 14 March 2005.
2. August Ferdinand Möbius
3. M.C. Escher, Mobius Strip I, 1961
4. M.C. Escher, Mobius Strip II, 1964
5. Roman Mosaic featuring Mobius Strip. Aion, the god of eternity, standing inside a celestial sphere. In front of him is the mother-earth goddess, Tellus.
6. Google Drive Logo
7. Gold Möbius Ring. Credit: Ka Gold Jewelry.
8. Möbelius Coffee Table by Deniz Aktay. Inspired by the möbius strip, the table top has a single continuous surface running along the table’s form.